Editor's note: To see masters, please click on each drawing.

The fine, intricately patterned latticework of diatom frustules and radiolarian tests rates as one of many natural aesthetic wonders. However, how and why these structures come into being, and why they form the patterns that they do, is a whole topic in itself.

Function

The holes in radiolarian and diatom shells respectively exist for differing reasons. Both types of skeleton are formed from silicon compounds.

In diatoms, the holes collectively take on the role of a sieve, a two-way filtration mechanism across which water and nutrient molecules permeate the cell. The holes can range from several micrometres down to 100 nanometres in diameter.

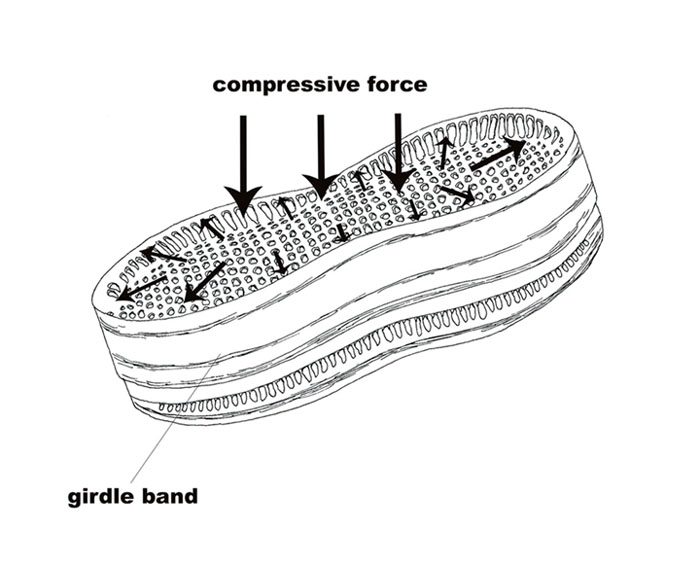
A typical diatom frustule is perforated rather than solid, for two reasons. Firstly, the perforated construction allows for a more economic use of silica, especially where low levels of dissolved silica are present. Silicon is also relatively dense, so the structure promotes lightness. Perforations in the frustule also endow the diatom with considerable compressive strength, which explains the frustules' ability to survive undamaged under layers of sediment. When compressive force is applied to a frustule, the lines of force are concentrated along the lines of the silica lattice and continued to the girdle band, which has a greater ability to withstand stress. Costae, or ribs, will also strengthen the upper and lower surfaces of the frustule.

In general, the perforations are termed areolae or porelli (in the case of a sieve membrane being present), or punctae (when no sieve membrane is present). Freshwater diatoms such as Gomphonema or Cymbella may have groups of porelli at their poles in an arrangement called an apical pore field. This accommodates mucilaginous stalks which the diatoms use to attach themselves to surfaces.

The numerous rows of areolae seen radiating from the centre of centric diatoms are collectively termed the fascicle.

In radiolarians, the holes (pores), larger and more irregular than in diatom frustules, allow axopods, retractable pseudopodia or fingerlike projections which catch food, to extrude. The shape of the test, roughly based on a sphere or system of spheres and ornamented with an arrangement of spines corresponding to pore patterning, allows the organism to drift with ocean currents and also makes for a considerable degree of buoyancy.

In theory, the skeletons of diatoms and radiolarians are also designed to offer protection against predators; however, dinoflagellates have evolved their own methods of circumventing this problem by engulfing or swallowing diatoms whole, or inserting a feeding tube into the gap between the two halves of the diatom frustule.

Formation

D'Arcy Thompson, revolutionary scientific thinker and author of the classic On Growth and Form, put forward the concept of vesicular formation of the frustule in the 1920s.

This proposed that the organism secreted a foam of closely packed vesicles around itself, in the interstices (termed Plateau borders and roughly positioned at 120° to each other) of which minerals from seawater were allowed to precipitate, gradually accreting to form the skeleton. This would lie on top of the vesicles, so that when skeletal formation was complete and the vesicles were reabsorbed, the result was a latticework of holes. To strengthen and stabilize the skeleton, silica would also be deposited in tubular vesicles positioned between the larger round areolar vesicles. Since then, D'Arcy's theory has indeed been proved to be correct; vesicles of polyamines are precipitated, around which micro-and nanostructured silica is deposited.

In some species of diatom, for example of the Coscinodiscus- or Stictodiscus- type, there appear to be holes of two or even three pore sizes. An examination of the frustules reveals that holes of a larger size accommodate perforations of a smaller size. This is due to the filling of the larger holes, once the main body of the exoskeleton is in place, with smaller vesicles arranged in secondary and /or tertiary lattices termed cribrum, which likewise deposit silica around themselves.

The smaller vesicles are produced from remnants of the larger bubbles as these disintegrate following deposition of the initial mesh.

An example of secondary areoles formed within spaces left by primary vesicle deposition.

Frustule shapes and pattern creation

The three-dimensional shape of the organism's frustule directly affects its two-dimensional surface. Depending on the organism's size and its complexity of form, the perforations take on a variety of different shapes and are arrayed according to a number of different schemes. In the above illustration, for example, the larger areoles take on a randomly sized hexagonal or pentagonal shape. This perhaps reflects the considerable curvature/large size of the frustule and exceptionally close packing of the template vesicles. The smaller holes can be observed to be more circular, since the smaller vesicles appear to have been positioned further apart and subject to less compression from, and distortion by, their neighbours.

Hexagonal areoles giving a “honeycomb” effect can be seen in the three-sided diatom Triceratium favus. Hexagonal holes are the result of the closest possible packing of vesicles, ideally resulting in a formation where each vesicle is surrounded by six others. It is interesting that, unlike the holes in similarly sized triangular diatoms, the perforations appear to be arranged in rows, rather than radiating from the centre; the fact that the diatom is three-sided does much to reinforce this illusion, and ensures that the “rows” will “read” correctly whichever side they are viewed from. A similar honeycomb effect is noticeable in the radiolarian Aulonia hexagona, and also in the centric diatom below it.

Aulonia hexagona, whose morphology was extensively studied by Ernst Haeckel.

This diatom, possibly Thalassiosira eccentrica, has an amazing three-dimensional shape. The example I drew was fairly small, approximately the same size as Triceratium pentacrinus, and seemed unremarkable at first glance. However, focusing up and down revealed an array of “areoles within areoles” and differently sized spines/processes.

I noticed that curvature can to some extent play a part in the formation of hexagonal holes in a honeycomb arrangement. The frustule of Triceratium favus, which is very gently curved, accommodates an almost perfect hexagonal arrangement. However, a polyhedral form which uses a sphere as its base can nevertheless not be completely closed by hexagonal shapes, as was demonstrated and reported by mathematician Leonhard Euler, biologist and artist Ernst Haeckel, and architect Richard Buckminster Fuller. In practice, at least 12 pentagons, and some square shapes or even heptagons, are needed to close a polyhedral form that otherwise consists of hexagons.

What the vesicle-deposition theory does not necessarily explain is why the holes occasionally take on more outlandish shapes. Square-shaped holes, neatly arranged in concentric rows after a pseudo-rectilinear fashion, make up the main patterning of the diatom below.

This large and very beautiful diatom has the appearance of a kaleidoscope interior. The top of the frustule undulates like a fan, and in my estimation, one half-frustule contains approx. 1500 holes. It was kindly identified by Rene van Wezel as a species of Arachnoidiscus (literally, "spider's web diatom").

A rectilinear orientation of pores is also present in this grenade-shaped radiolarian, possibly a member of the Artostobiidae:

Broadly speaking, where close-packing of large vesicles has been present, it seems that curved surfaces favour circular holes and a hexagonal arrangement, whereas flatter surfaces, or forms with a strong unidirectional dimension or orientation, favour rectangular areoles arranged in rectilinear rows. Therefore, the shape of the perforations can show some consistency according to the three-dimensional form of the frustule. Both types of areole may be present in one organism.

The radiolarian Thyrsocyrtis tensa, showing a hexagonal arrangement of holes. Compare it to the radiolarian above it, whose rectilinear arrangement of pores perhaps derives from its more cylindrical (and therefore more unidirectional) form.

Where extreme close-packing or large vesicles have obviously not been present, the holes tend to be much reduced in size, and either be randomly scattered throughout the frustule, radiate from the centre, or lie in accordance with the lines of patterned ridging or protuberances present on the top surface of the frustule.

In this diatom, possibly Auliscus sculptus, the positioning of the holes follows the ridging on the top of the frustule. The holes are much reduced in size.

Lastly, in this radiolarian of the Stylatractus group, the pores take on a shape similar to a five-petalled flower! The assumption I made here was that the shape was due to clusters of up to 6 vesicles, which group together in a close-packed formation, resulting in the “flower” shape.

This proposed method of areole formation also raises the question of how closely diatoms and radiolarians are related.

****************************************************************************************

Drawings are from slides supplied by Klaus Kemp, and Brian Darnton.

Klaus Kemp's website: http://www.diatoms.co.uk

Brian Darnton's homepage: http://www.darnton.freeserve.co.uk

Literary sources:

The Self-Made Tapestry - Pattern formation in nature, Philip Ball, publ. Oxford University Press, 2001 (paperback) ISBN: 0 19 850243 5

On Growth and Form, D'Arcy Thompson, publ. Cambridge University Press (Canto edition), 2004 (paperback) ISBN: 0 521 43776 8

Web sources:

http://www.calacademy.org/research/diatoms/overview/glossary.html

Visit Christina Brodie's website for contact details and to see more of her stunning botanical and natural history drawings and paintings: www.queen-christina.com .

Note: Some of the drawings of diatoms featured in this article will be exhibited as part of the botanical artwork exhibition hosted by the RHS at BBC Gardeners' World Live at Birmingham NEC in June.

Images © Christina Brodie 2005. All rights reserved.

 

Editor's note: Christina Brodie has shared a selection of her other beautiful drawings of nature, including artwork of microscopic organisms, in the Micscape articles below: